Projectile Motion

Learning Objectives. Identify and explain the properties of a projectile, such as acceleration due to gravity, range, maximum height, and trajectory.

‪Projectile Motion‬ - PhET Interactive Simulations. The projectile motion calculator is an online tool which helps you examine the parabolic projectile motion. It provides different values which is why it’s also known as a horizontal distance calculator, maximum height calculator or kinematic calculator.

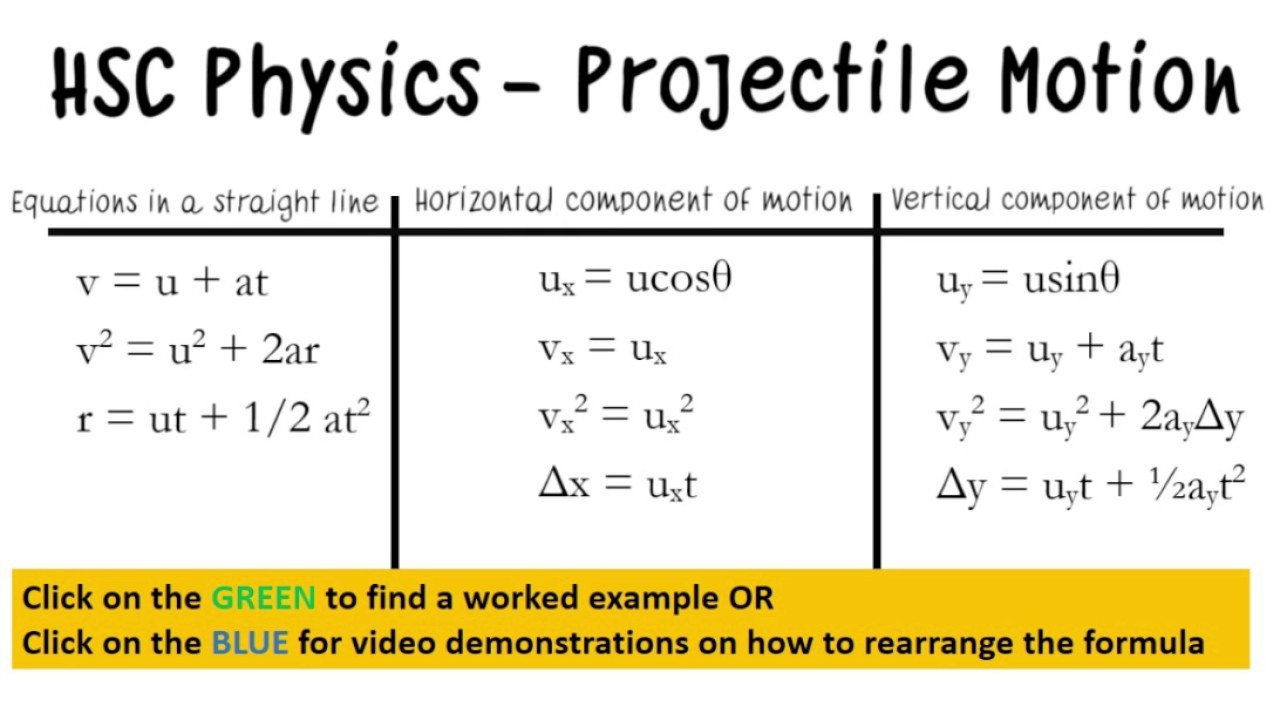
Determine the location and velocity of a projectile at different points in its trajectory. Apply the principle of independence of motion to solve projectile motion problems.Projectile motion is the motion of an object thrown or projected into the air, subject to only the acceleration of gravity. The object is called a projectile, and its path is called its trajectory. The motion of falling objects, as covered in, is a simple one-dimensional type of projectile motion in which there is no horizontal movement. In this section, we consider two-dimensional projectile motion, such as that of a football or other object for which air resistance is negligible.The most important fact to remember here is that motions along perpendicular axes are independent and thus can be analyzed separately. This fact was discussed in, where vertical and horizontal motions were seen to be independent. The key to analyzing two-dimensional projectile motion is to break it into two motions, one along the horizontal axis and the other along the vertical.

(This choice of axes is the most sensible, because acceleration due to gravity is vertical—thus, there will be no acceleration along the horizontal axis when air resistance is negligible.) As is customary, we call the horizontal axis the x-axis and the vertical axis the y-axis. Illustrates the notation for displacement, where is defined to be the total displacement and and are its components along the horizontal and vertical axes, respectively.

The magnitudes of these vectors are s, x, and y. (Note that in the last section we used the notation to represent a vector with components.

If we continued this format, we would call displacement with components. However, to simplify the notation, we will simply represent the component vectors as and.)Of course, to describe motion we must deal with velocity and acceleration, as well as with displacement.

While Picross presents a rectangular grid of squares, which must be filled in to create a picture, Picross 3D uses a rectangular prism made of a number of smaller cubes, and must be chipped away in order to construct an image in three dimensions. Each row or column has at most one number corresponding to it, and many do not have any numbers at all; the number indicates how many cubes the row/column should contain when the picture is complete. Picross 3D, known in Japan as Rittai Picross, is a puzzle video game developed by HAL Laboratory and published by Nintendo for the Nintendo DS. It was released in Japan in March 2009, in Europe in March 2010, and in North America in May 2010. It uses similar nonogram mechanics to Picross DS, but it puts it in 3D. Outside Japan, the game is part of Nintendo's Touch! Generations brand. A sequel, Picross 3D: Round 2, was released for the Nintendo 3DS in Japan in October 2015, in North America in Se. The experiment was a success and Picross 3D is another addictive collection of satisfying mind games. Only the visuals, which took a dramatic turn for the worse, keep this sequel from sitting. Picross 3d collection. Picross 3D lets players create their own customized puzzles. Players can either share their creations with other Picross 3D players via a local wireless connection or use their broadband Internet connection and Nintendo Wi-Fi Connection to submit their puzzles into a monthly themed puzzle challenge.

We must find their components along the x– and y-axes, too. We will assume all forces except gravity (such as air resistance and friction, for example) are negligible. The components of acceleration are then very simple. (Note that this definition assumes that the upwards direction is defined as the positive direction. If you arrange the coordinate system instead such that the downwards direction is positive, then acceleration due to gravity takes a positive value.) Because gravity is vertical. Both accelerations are constant, so the kinematic equations can be used.

The total displacement of a soccer ball at a point along its path. The vector has components and along the horizontal and vertical axes. Its magnitude is, and it makes an angle with the horizontal.Given these assumptions, the following steps are then used to analyze projectile motion:Step 1. Resolve or break the motion into horizontal and vertical components along the x- and y-axes. These axes are perpendicular, soandare used.

The magnitude of the components of displacementalong these axes are andThe magnitudes of the components of the velocity areandwhereis the magnitude of the velocity and is its direction, as shown in. Initial values are denoted with a subscript 0, as usual.Step 2. Treat the motion as two independent one-dimensional motions, one horizontal and the other vertical. The kinematic equations for horizontal and vertical motion take the following forms. Solve for the unknowns in the two separate motions—one horizontal and one vertical. Note that the only common variable between the motions is time. The problem solving procedures here are the same as for one-dimensional kinematics and are illustrated in the solved examples below.Step 4.

Recombine the two motions to find the total displacement and velocity. Because the x – and y -motions are perpendicular, we determine these vectors by using the techniques outlined in the and employingand in the following form, where is the direction of the displacement and is the direction of the velocity:Total displacement and velocity. (a) We analyze two-dimensional projectile motion by breaking it into two independent one-dimensional motions along the vertical and horizontal axes. (b) The horizontal motion is simple, because and is thus constant. (c) The velocity in the vertical direction begins to decrease as the object rises; at its highest point, the vertical velocity is zero. As the object falls towards the Earth again, the vertical velocity increases again in magnitude but points in the opposite direction to the initial vertical velocity. (d) The x – and y -motions are recombined to give the total velocity at any given point on the trajectory.

A Fireworks Projectile Explodes High and AwayDuring a fireworks display, a shell is shot into the air with an initial speed of 70.0 m/s at an angle of above the horizontal, as illustrated in. The fuse is timed to ignite the shell just as it reaches its highest point above the ground.

(a) Calculate the height at which the shell explodes. (b) How much time passed between the launch of the shell and the explosion? (c) What is the horizontal displacement of the shell when it explodes?StrategyBecause air resistance is negligible for the unexploded shell, the analysis method outlined above can be used. The motion can be broken into horizontal and vertical motions in which. We can then defineand to be zero and solve for the desired quantities.Solution for (a)By “height” we mean the altitude or vertical position above the starting point. The highest point in any trajectory, called the apex, is reached when. Since we know the initial and final velocities as well as the initial position, we use the following equation to find.

Discussion for (a)Note that because up is positive, the initial velocity is positive, as is the maximum height, but the acceleration due to gravity is negative. Note also that the maximum height depends only on the vertical component of the initial velocity, so that any projectile with a 67.6 m/s initial vertical component of velocity will reach a maximum height of 233 m (neglecting air resistance).

The numbers in this example are reasonable for large fireworks displays, the shells of which do reach such heights before exploding. In practice, air resistance is not completely negligible, and so the initial velocity would have to be somewhat larger than that given to reach the same height.Solution for (b)As in many physics problems, there is more than one way to solve for the time to the highest point. In this case, the easiest method is to use.

Because is zero, this equation reduces to simply. Defining a Coordinate SystemIt is important to set up a coordinate system when analyzing projectile motion. One part of defining the coordinate system is to define an origin for the and positions. Often, it is convenient to choose the initial position of the object as the origin such that. It is also important to define the positive and negative directions in the and directions.

Typically, we define the positive vertical direction as upwards, and the positive horizontal direction is usually the direction of the object’s motion. When this is the case, the vertical acceleration, takes a negative value (since it is directed downwards towards the Earth). However, it is occasionally useful to define the coordinates differently. For example, if you are analyzing the motion of a ball thrown downwards from the top of a cliff, it may make sense to define the positive direction downwards since the motion of the ball is solely in the downwards direction.

If this is the case, takes a positive value. Calculating Projectile Motion: Hot Rock ProjectileKilauea in Hawaii is the world’s most continuously active volcano. Very active volcanoes characteristically eject red-hot rocks and lava rather than smoke and ash.

Suppose a large rock is ejected from the volcano with a speed of 25.0 m/s and at an angle above the horizontal, as shown in. The rock strikes the side of the volcano at an altitude 20.0 m lower than its starting point.

(a) Calculate the time it takes the rock to follow this path. (b) What are the magnitude and direction of the rock’s velocity at impact? The trajectory of a rock ejected from the Kilauea volcano.StrategyAgain, resolving this two-dimensional motion into two independent one-dimensional motions will allow us to solve for the desired quantities.

The time a projectile is in the air is governed by its vertical motion alone. We will solve for first.

While the rock is rising and falling vertically, the horizontal motion continues at a constant velocity. This example asks for the final velocity. Thus, the vertical and horizontal results will be recombined to obtain and at the final time determined in the first part of the example.Solution for (a)While the rock is in the air, it rises and then falls to a final position 20.0 m lower than its starting altitude.

We can find the time for this by using. Discussion for (a)The time for projectile motion is completely determined by the vertical motion. So any projectile that has an initial vertical velocity of 14.3 m/s and lands 20.0 m below its starting altitude will spend 3.96 s in the air.Solution for (b)From the information now in hand, we can find the final horizontal and vertical velocities and and combine them to find the total velocity and the angle it makes with the horizontal. Of course, is constant so we can solve for it at any horizontal location. In this case, we chose the starting point since we know both the initial velocity and initial angle.

Discussion for (b)The negative angle means that the velocity is below the horizontal. This result is consistent with the fact that the final vertical velocity is negative and hence downward—as you would expect because the final altitude is 20.0 m lower than the initial altitude.

(See.)One of the most important things illustrated by projectile motion is that vertical and horizontal motions are independent of each other. Galileo was the first person to fully comprehend this characteristic. He used it to predict the range of a projectile. On level ground, we define range to be the horizontal distance traveled by a projectile. Galileo and many others were interested in the range of projectiles primarily for military purposes—such as aiming cannons.

However, investigating the range of projectiles can shed light on other interesting phenomena, such as the orbits of satellites around the Earth. Let us consider projectile range further. Trajectories of projectiles on level ground. (a) The greater the initial speed, the greater the range for a given initial angle. (b) The effect of initial angle on the range of a projectile with a given initial speed. Note that the range is the same for and, although the maximum heights of those paths are different.How does the initial velocity of a projectile affect its range?

Obviously, the greater the initial speed, the greater the range, as shown in (a). The initial angle also has a dramatic effect on the range, as illustrated in (b). For a fixed initial speed, such as might be produced by a cannon, the maximum range is obtained with. This is true only for conditions neglecting air resistance. If air resistance is considered, the maximum angle is approximately. Interestingly, for every initial angle except, there are two angles that give the same range—the sum of those angles is.

The range also depends on the value of the acceleration of gravity. The lunar astronaut Alan Shepherd was able to drive a golf ball a great distance on the Moon because gravity is weaker there. The range of a projectile on level ground for which air resistance is negligible is given.

Where is the initial speed and is the initial angle relative to the horizontal. The proof of this equation is left as an end-of-chapter problem (hints are given), but it does fit the major features of projectile range as described.When we speak of the range of a projectile on level ground, we assume that is very small compared with the circumference of the Earth.

If, however, the range is large, the Earth curves away below the projectile and acceleration of gravity changes direction along the path. The range is larger than predicted by the range equation given above because the projectile has farther to fall than it would on level ground. (See.) If the initial speed is great enough, the projectile goes into orbit. This possibility was recognized centuries before it could be accomplished. When an object is in orbit, the Earth curves away from underneath the object at the same rate as it falls.

The object thus falls continuously but never hits the surface. These and other aspects of orbital motion, such as the rotation of the Earth, will be covered analytically and in greater depth later in this text.Once again we see that thinking about one topic, such as the range of a projectile, can lead us to others, such as the Earth orbits. In, we will examine the addition of velocities, which is another important aspect of two-dimensional kinematics and will also yield insights beyond the immediate topic. Summary. Projectile motion is the motion of an object through the air that is subject only to the acceleration of gravity.

To solve projectile motion problems, perform the following steps:. Determine a coordinate system. Then, resolve the position and/or velocity of the object in the horizontal and vertical components. The components of position are given by the quantities and, and the components of the velocity are given by and, where is the magnitude of the velocity and is its direction.

Analyze the motion of the projectile in the horizontal direction using the following equations. An archer shoots an arrow at a 75.0 m distant target; the bull’s-eye of the target is at same height as the release height of the arrow. (a) At what angle must the arrow be released to hit the bull’s-eye if its initial speed is 35.0 m/s? In this part of the problem, explicitly show how you follow the steps involved in solving projectile motion problems. (b) There is a large tree halfway between the archer and the target with an overhanging horizontal branch 3.50 m above the release height of the arrow.

Will the arrow go over or under the branch? The cannon on a battleship can fire a shell a maximum distance of 32.0 km. (a) Calculate the initial velocity of the shell. (b) What maximum height does it reach? (At its highest, the shell is above 60% of the atmosphere—but air resistance is not really negligible as assumed to make this problem easier.) (c) The ocean is not flat, because the Earth is curved.

Assume that the radius of the Earth is. How many meters lower will its surface be 32.0 km from the ship along a horizontal line parallel to the surface at the ship? Does your answer imply that error introduced by the assumption of a flat Earth in projectile motion is significant here? The free throw line in basketball is 4.57 m (15 ft) from the basket, which is 3.05 m (10 ft) above the floor. A player standing on the free throw line throws the ball with an initial speed of 7.15 m/s, releasing it at a height of 2.44 m (8 ft) above the floor.

At what angle above the horizontal must the ball be thrown to exactly hit the basket? Note that most players will use a large initial angle rather than a flat shot because it allows for a larger margin of error. Explicitly show how you follow the steps involved in solving projectile motion problems. Construct Your Own Problem Consider a ball tossed over a fence. Construct a problem in which you calculate the ball’s needed initial velocity to just clear the fence. Among the things to determine are; the height of the fence, the distance to the fence from the point of release of the ball, and the height at which the ball is released. You should also consider whether it is possible to choose the initial speed for the ball and just calculate the angle at which it is thrown.

Also examine the possibility of multiple solutions given the distances and heights you have chosen.

Introduction

In this lab you will study the motion of a freely-falling projectile, namely a small plastic sphere. Projectile motion, for our purposes, is the motion of an object that has been launched and then is subject to only the force of gravity and the force of air friction. The Newtonian mechanics principles that you have been studying allow you to predict this type of motion quite well. You will perform two experiments to aid your understanding of these principles, which will be described later in the lab. Since there is the small but real possibility of causing injury to yourself or another person, please follow all safety guidelines and common sense safety rules.

Time-of-flight vs. Initial Velocity

The purpose of this experiment is to determine whether the time-of-flight of a ball launched horizontally off the table varies as the initial velocity is varied.A ball launched horizontally from a table of height h has no initial velocity in the vertical direction, so the ball should take the same amount of time to reach the ground as a ball that drops from rest from the same height. The kinematic equation can be used to determine the time-of-flight, which is independent of initial velocity:t =

Figure 1

Projectile Motion

The purpose of this experiment is to predict and verify the range and the time-of-flight of a projectile launched at an angle.

Figure 2

To predict the range of the projectile when it is shot off a table at some angle above the horizontal, it is necessary first to determine the initial speed (muzzle velocity) of the ball. The initial velocity of the ball is determined by shooting it, at the appropriate angle, through 2 photogates that are placed near the muzzle and only a few centimeters apart from each other. Then the initial velocity can be used to calculate where the ball will land when it is shot at some angle θ.Initial velocity: The photogates are approximately 10 centimeters apart (measure directly to confirm this). A Smart Timer can be used to measure the time the ball takes to travel between these two gates. The average speed between the gates can then be calculated from v = (10 cm)/time.Time-of-flight and range: To predict the total time-of-flight, you can use the vertical y-component of the initial velocity along with the initial and final y-coordinates of the ball. To predict the range, you can use the total time-of-flight and the x-component of the initial velocity.You will derive these two equations, one for the range and one for the total time-of-flight, before you actually perform the experiment. Then, you will calculate values for the range and time-of-flight using your equations. After you calculate the expected values, you will perform the experiment to see if you calculated correctly!

Procedure

General Operation of the Projectile Launcher

Caution:
Safety glasses must be worn during this experiment.

Caution:
When the projectile launcher is loaded, a yellow indicator is visible in one of the range slots in the side of the barrel and the ball is visible in another one of the slots in the side of the barrel. As with all projectile launching mechanisms, NEVER LOOK DOWN THE BARREL WHEN IT IS LOADED. To check to see if the launcher is loaded, always check the side of the barrel.

Before shooting the ball, make certain no one is in its flight path. To shoot the ball, pull straight up on the string that is attached to the trigger. It is only necessary to pull it about a centimeter.

Time-of-flight vs. Initial Velocity

Equipment Set-Up

The launchers should be set up when you arrive; do not adjust the placement of the launchers unless instructed to do so by your TA. Each launcher should be clamped to the edge of a lab bench and aimed so that the ball will land on the floor without hitting any other lab groups.

Adjust the angle of the projectile launcher to zero degrees (0°).
2
Connect the lead from the photogate closest to the muzzle of the launcher into port 1 on the right side of the Smart Timer.
Plug the time-of-flight plate into port 2 of the Smart Timer.
4
Turn on the Smart Timer and select Time and Two Gates mode. Press the Start button on the Smart Timer, and an asterisk (*) should appear indicating that the device is ready to collect data. Now, as the ball leaves the muzzle of the launcher, it signals the timer to start timing when it passes through the first gate. When it lands on the time-of-flight plate, a second signal is sent to the timer that tells it to stop. The time recorded is the time-of-flight.

Note: If the timer does not start, the photogate beam may be blocked by the launcher, in which case the bracket should be moved outward so that the first photogate is just beyond the front end of the launcher.

Time-of-Flight

Put on your safety glasses.
2
Measure the vertical distance from the bottom of the ball's launch position in the barrel (this position is marked on one side of the barrel) to the top of the strike plate.
Put the yellow plastic ball into the projectile launcher and cock it to the short range position.
4
Test fire the ball to determine where to place the time-of-flight plate. Put the time-of-flight plate on the floor where the ball lands. Make sure it hits ONLY in the white area on the plate and that the path of the ball is parallel to the longest side of the white area. Practice and patience are required to ensure that the ball accurately lands on the pad and the time of flight is properly recorded.

Whenever you launch a ball, position one member of your lab group ready to catch the ball after it lands to avoid losing the ball or interfering with other students in the room.

Fire five shots and record the time-of-flight for each trial. Remember to push the Start/Stop button on the photogate timer before firing.
6
Measure and record the horizontal distance (range) traveled by the ball.
Repeat steps 3 to 6 for the medium range launch position.

You should observe that the time of flight does not depend on the initial velocity when the ball is launched horizontally. Calculate the initial velocity for each of the two launch settings from

v_o = Δx/Δt,

where

Δx is the range or horizontal displacement of the ball.

Projectile Motion

Measuring the Initial Velocity Directly

Set the angle of the launcher to 0°.
2
Disconnect the time-of-flight plate from the Smart Timer, and connect the second photogate in port 2 so that the timer will now record the time for the ball to pass between the two gates.
Load the ball into the short range setting, reset the timer, and launch the ball. Record the time taken for the ball to travel between the gates.
4
Calculate the initial velocity, v_o, using the distance and time between the gates.
Repeat steps 3 and 4 several times and calculate the average initial velocity and uncertainty.

Predicting and Verifying the Range and Total Time-of-Flight

Use the equations you derived in the Pre-lab Assignment to calculate the expected range and time-of-flight using your best estimate of the average initial velocity for the short range setting, and the launch angle. To test your predictions, follow the steps outlined below.

1
Adjust the angle of the launcher to 30 degrees. Use a binder clip to hold a piece of paper to the time-of-flight pad, and place a piece of carbon paper (carbon side down) on top. Place the time-of-flight pad at the spot you predict the ball to land. You will also want to record the time-of-flight: unplug the second gate from the Smart Timer and plug in the cord from the time-of-flight pad.
Test fire the ball. If you miss the time-of-flight pad, check your calculations and try again!
3
Launch the ball five times at 30°, and record the time-of-flight each time. To find the range for each trial, use a plumb bob to find the point on the floor that is directly beneath the release point of the ball marked on the barrel of the launcher (there is a diagram of the ball on the side of the launcher that shows the release point). Measure the horizontal distance from the point on the floor beneath the release point to each of the five landing points. If you need to move the plate between launches, remember to record the necessary range values first!

Target Challenge (optional)

For an additional challenge, your TA may place a target or basket at a specified point for you to try to hit. Use your equations to determine an appropriate launch setting to score a hit!

Analysis

Time-of-flight vs. Initial Velocity

Calculate the average time-of-flight and the uncertainty for the short and medium ranges.
2
Calculate the average initial velocity for the short and medium ranges.

Projectile Motion

Calculate the average initial velocity for the short range using the two photogates. Does this value agree with that found in Time-of-flight vs. Initial Velocity? Which method do you believe is more accurate?
2
Compare your predicted and measured ranges and flight times. Do they agree within the experimental uncertainties? If not, explain why there is a discrepancy.

Discussion

Did the time-of-flights for part one change with the initial velocity? Discuss the differences between your predicted and experimental results for both the range and time-of-flight. Is there agreement to within the uncertainties? If not, explain. Which is more significant for this lab: random or systematic errors? How can you tell? What do you believe is the primary source of uncertainty in this experiment? What would you do differently to improve your results? How significant is air resistance for this experiment? Use your experimental results to estimate the maximum relative error introduced by this factor.

A third group that inhabits Clardona are spirits, entities from another world, which mainly manifest in the form of nature spirits, and rarely as creatures or humanoids known as Summon Beasts. Humans can call up creatures from another world in a process known as 'Summoning' and they are constantly researching ways to increase their summoning powers. In one of the facilities, a terrible accident happened caused by a young girl named Reiha's summoning powers getting out of control. Summon night twin age cheat codes. The girl was assumed to have died along with her family in the accident, but she survived, and was hidden with the Kascuza on the island of Jarazi, along with the result of the summoning, a young Summon Beast boy named Aldo. The two races are constantly battling, and a short-lived peace began after the humans pushed the Kascuza onto a small island called Jarazi.